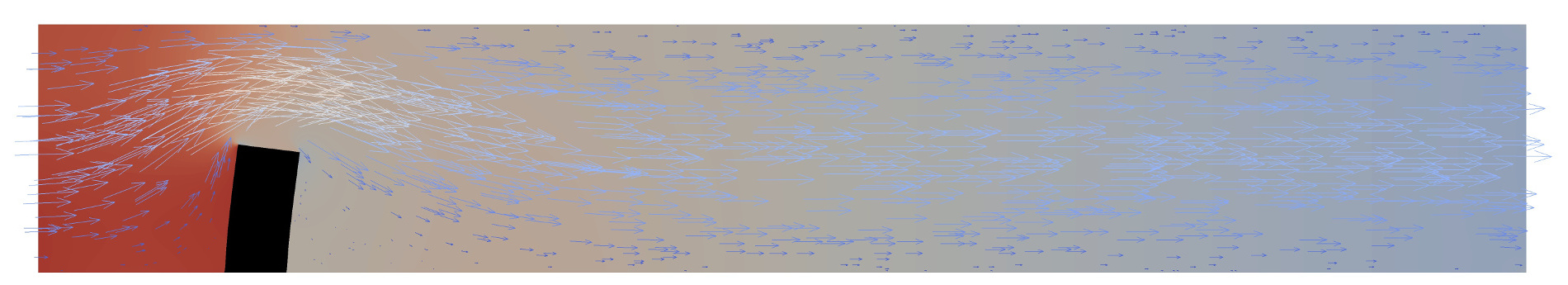
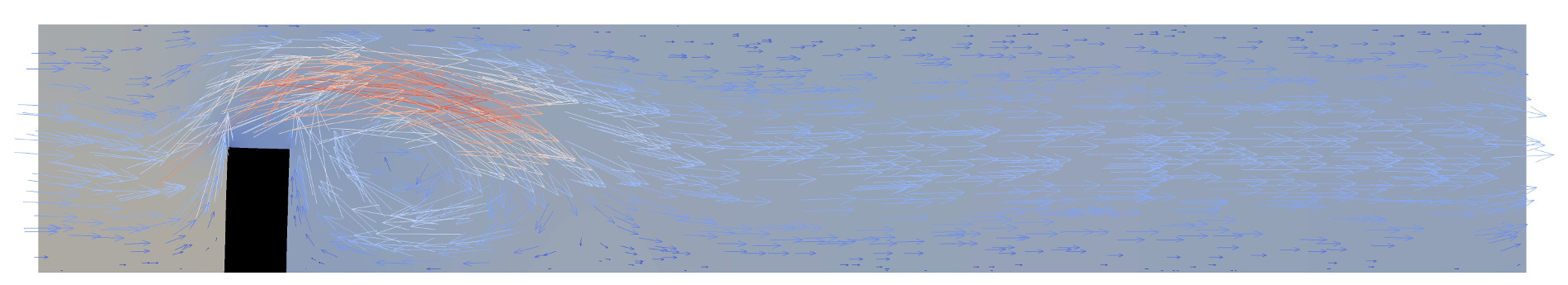
The main topic of my PhD project involves finding fast methods to approximate solutions to parameter-dependent fluid-structure interaction problems. Such approximations show the behavior of a fluid-structure interaction model for different solid and fluid configurations.
Fluid-structure interaction problems can be described by systems of partial differential equations (PDEs) that are defined on a fluid, a solid domain and an interaction interface. In natural science, partial differential equations are used to describe physical processes such as water flow or the behavior of a solid. Discretizations of fluid-structure interaction problems are challenging since the number of degrees of freedom is typically very high and the resulting system matrices are not symmetric. In addition, such PDE systems are often highly nonlinear if one considers, for instance, a model that uses the arbitrary Lagrangian Eulerian (ALE) formulation. Parameter-dependent discretization leads to multiple equation systems that often depend nonlinearly on the unknown. The unknown is then a matrix instead of a vector. Here, low-rank methods can be applied to approximate this matrix in a fast and storage-saving way. The approximation is represented as a tensor of low rank. Such a representation reduces the complexity of operations applied to the approximation and therefore the complexity of the whole method.